How To Find N In Pv Nrt
| Ideal GASES AND THE Platonic GAS Law This page looks at the assumptions which are fabricated in the Kinetic Theory near platonic gases, and takes an introductory wait at the Ideal Gas Constabulary: pV = nRT. This is intended only as an introduction suitable for chemistry students at virtually UK A level standard (for 16 - eighteen year olds), and then there is no endeavor to derive the ideal gas police using physics-style calculations. Kinetic Theory assumptions almost ideal gases There is no such thing as an ideal gas, of course, but many gases bear approximately as if they were platonic at ordinary working temperatures and pressures. Real gases are dealt with in more item on some other page. The assumptions are:
And then two absolutely key assumptions, considering these are the two most important means in which existent gases differ from ideal gases:
The Platonic Gas Equation The ideal gas equation is: pV = nRT On the whole, this is an easy equation to remember and use. The problems lie almost entirely in the units. I am assuming below that y'all are working in strict SI units (as you will be if you are doing a Great britain-based examination, for example). Exploring the various terms Pressure, p Pressure is measured in pascals, Pa - sometimes expressed every bit newtons per square metre, N m-2. These mean exactly the same thing. Be careful if you are given pressures in kPa (kilopascals). For example, 150 kPa is 150000 Pa. You must brand that conversion before you use the platonic gas equation. Should you lot want to convert from other pressure measurements:
Volume, Five This is the most likely identify for you to get wrong when you use this equation. That'south because the SI unit of book is the cubic metre, m3 - not cm3 or dm3. 1 mthree = 1000 dm3 = 1 000 000 cmiii So if you are inserting values of book into the equation, you lot first have to convert them into cubic metres. Y'all would have to split a volume in dm3 by thou, or in cmiii by a million. Similarly, if you are working out a volume using the equation, remember to covert the respond in cubic metres into dm3 or cm3 if yous need to - this time past multiplying past a 1000 or a million. If you get this wrong, you are going to cease up with a silly answer, out by a cistron of a one thousand or a one thousand thousand. And so it is normally fairly obvious if you lot have washed something incorrect, and you can check dorsum again. Number of moles, due north This is like shooting fish in a barrel, of course - it is but a number. Yous already know that you work it out by dividing the mass in grams past the mass of one mole in grams. You volition most often apply the ideal gas equation by first making the substitution to requite: I don't recommend that you recall the ideal gas equation in this form, but you must be confident that you can convert it into this form. The gas constant, R A value for R will be given you if you demand it, or you can look it upwardly in a information source. The SI value for R is eight.31441 J G-one mol-1. | |
| Note:You may come across other values for this with different units. A usually used one in the past was 82.053 cmiii atm K-i mol-1. The units tell you that the book would be in cubic centimetres and the pressure level in atmospheres. Unfortunately the units in the SI version aren't and so obviously helpful. | |
| The temperature, T The temperature has to be in kelvin. Don't forget to add 273 if you are given a temperature in degrees Celsius. Using the platonic gas equation Calculations using the ideal gas equation are included in my calculations book (see the link at the very lesser of the page), and I can't repeat them here. There are, still, a couple of calculations that I haven't washed in the volume which give a reasonable idea of how the ideal gas equation works. The molar volume at stp If you have done simple calculations from equations, you have probably used the molar volume of a gas. ane mole of any gas occupies 22.4 dm3 at stp (standard temperature and pressure, taken as 0°C and 1 atmosphere force per unit area). You may besides take used a value of 24.0 dm3 at room temperature and pressure (taken equally about 20°C and 1 atmosphere). These figures are really just true for an ideal gas, and we'll have a look at where they come from. We can utilize the ideal gas equation to calculate the volume of 1 mole of an ideal gas at 0°C and 1 atmosphere pressure. First, we have to go the units correct. 0°C is 273 Thousand. T = 273 K ane atmosphere = 101325 Pa. p = 101325 Pa We know that due north = ane, considering we are trying to calculate the volume of 1 mole of gas. And, finally, R = viii.31441 J K-1 mol-1. Slotting all of this into the ideal gas equation and so rearranging it gives: And finally, because we are interested in the volume in cubic decimetres, you have to remember to multiply this past 1000 to convert from cubic metres into cubic decimetres. The molar volume of an platonic gas is therefore 22.4 dm3 at stp. And, of course, you could redo this calculation to observe the volume of 1 mole of an ideal gas at room temperature and pressure level - or any other temperature and pressure. Finding the relative formula mass of a gas from its density This is nearly every bit tricky as it gets using the platonic gas equation. The density of ethane is i.264 g dm-3 at twenty°C and i atmosphere. Calculate the relative formula mass of ethane. The density value means that 1 dm3 of ethane weighs 1.264 g. Again, before nosotros exercise anything else, get the awkward units sorted out. A pressure of 1 atmosphere is 101325 Pa. The volume of 1 dmiii has to be converted to cubic metres, by dividing by thou. We have a book of 0.001 m3. The temperature is 293 G. At present put all the numbers into the form of the ideal gas equation which lets you piece of work with masses, and rearrange it to work out the mass of 1 mole. The mass of 1 mole of anything is simply the relative formula mass in grams. And so the relative formula mass of ethane is 30.4, to 3 sig figs. Now, if you add up the relative formula mass of ethane, CtwoHhalf dozen using authentic values of relative atomic masses, yous go an answer of 30.07 to 4 significant figures. Which is dissimilar from our answer - so what's incorrect? In that location are ii possibilities.
If you need to know about real gases, now is a adept fourth dimension to read well-nigh them. Questions to test your understanding If this is the first set of questions you have washed, please read the introductory folio before you start. You will need to use the BACK BUTTON on your browser to come dorsum here afterward. questions on platonic gases answers
To explore real gases . . . To the kinetic theory menu . . . To the Concrete Chemistry menu . . . To Main Menu . . . © Jim Clark 2010 (concluding modified July 2017) | |
Source: https://www.chemguide.co.uk/physical/kt/idealgases.html

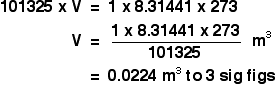

0 Response to "How To Find N In Pv Nrt"
Post a Comment